Tractive Effort (TE) and Tractive Resistance (TR)

Tractive effort (TE):

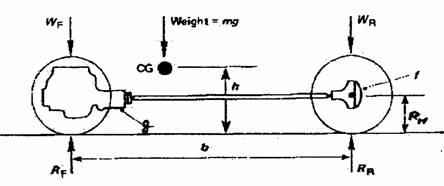
If the rear wheels of the vehicle in the figure are driven with no slip taking place between the tires and the level road surface, the wheel force or the tractive effort (TE) is equal to the torque at the driven wheels (T_w) divided by the rolling radius or effective radius (R_w)

T_w = T_e i_g i_f h_t   [N m]

and

TE =T_w / R_w  [N]

where:

T_w is the wheel torque [N m]

T_e is the engine torque [N m]

i_g is the gearbox ratio

i_f is the final drive ratio

h_t is the transmission efficiency

TE is the tractive effort [N]

R_w is the rolling radius or effective radius [m]

Maximum value of TE:

If the rear wheels have such a torque applied to them that they are on the point of slipping in the plane of the wheel, then the value of adhesive force between tire and road surface has been reached (φ W_R), where φ is the coefficient of adhesion between tire and road surface, and W_R is the weight on the rear wheels. Under these conditions the engine torque has reached the maximum useful value for the present set of conditions, as any increase would only result in slip and lower acceleration. Static friction is higher than kinetic friction.

Maximum road speed:

When tractive effort TE is equal to the tractive resistance TR a vehicle would be either stationary or moving at a uniform velocity. It the tractive effort exceeds the resistance the vehicle will accelerate until the tractive effort once again equals the tractive resistance. It follows that if the tractive resistance becomes greater than the tractive effort, the vehicle will decelerate and come to rest unless the tractive effort is increased or the resistance reduced.

Maximum possible road speed, [Maximum (maximum road speed)]

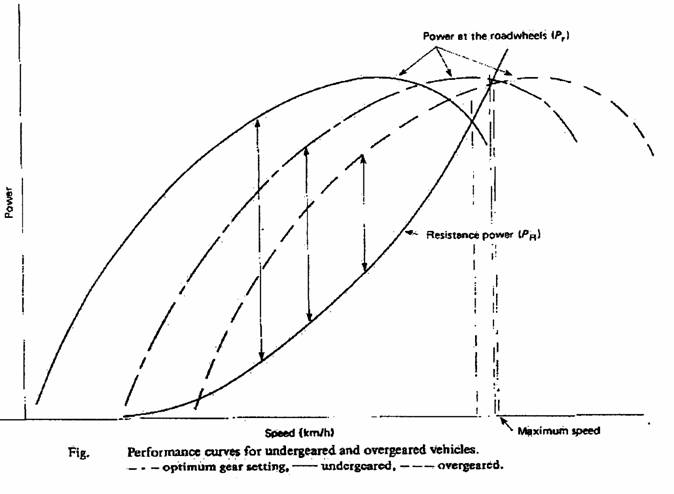
The maximum road speed of a vehicle in top gear on a level road is dependent upon the rolling and air resistance, the maximum power at the road wheels and the choice of a suitable final drive ratio.

Performance curves of resistance power (P_R) and power at the road wheels (P_r) are plotted on a km/h base. The relationship of one to the other will ascertain whether the vehicle is over geared or under geared. The optimum final drive setting will ensure that the maximum power available P_r crosses the resistance power curve P_R to give the maximum possible road speed as shown in the figure. If the power curve P_r is moved to the left relative to the resistance curve P_R the maximum road speed will be reduced, but more power becomes available for acceleration, etc., and the vehicle is termed ‘under geared’. The vertical distance between the two curves shows the power available for acceleration. If the P_r curve is moved to the right of the optimum setting, maximum road speed will be reduced together with power available for acceleration and the vehicle is ‘over geared’. These graphs, together with other information, are required before the final drive ratio and gearbox ratios for the vehicle can be decided.

Data and methods for setting final drive and gearbox ratios

Final drive ratio

Figures for power at the road wheels are calculated for the road speed range, and resistance power calculated for level road conditions using both rolling and air resistance data. The graphs are plotted on a km/h base. The exact location of the curves relative to each other will provide under gearing or over gearing conditions. The point where the power available at the road wheels crosses the resistance power denotes maximum road speed, and it will be noticed that a slight under gearing reduces the maximum road speed by a very small amount, as shown in the figure, but the increase in surplus tractive effort, and therefore power, more than compensates for the speed loss by providing good acceleration and flexibility.

Setting the final drive ratio (i_f):

The graphs and/or data are used to determine the engine speed at maximum road speed in the following formula for calculating the final drive.

Let us assume that the vehicle is designed to have a maximum speed of(v km/h at direct drive.

V_max =2 π R_w  (N_w)_max x (60/1000) = 0.377 R_w (N_w)_max

and

(N_w)_max = v_max / (0.377 R_w)

This (N_w)_max will occurs at the consonance engine speed at maximum engine power (N_e)_{p max}.

The relationship between the two speeds is:

(N_w)_max = (N_e)_{p max} / (i_g i_f),

We have i_g = 1, since the vehicle is moving at direct drive, then;

(N_w)_max = (Ne)_{p max} / i_f,

from this relation we conclude:

i_f = (N_e)_{p max} / (N_w)_{p max} = [(N_e)_{p max} (0.377 R )] / v_max

Worked example:

A vehicle is to have a maximum road speed of 150 km/h. If the engine develops its peak power at 6000 rev/mm and the effective road diameter is 0.54 m, determine the final drive gear ratio.

Solution:

i_f = [(N_e)_{p max} (0.377 R )] / v_max

   = (0.377 x (0.54 / 2) x 6000)/ 150 = 4.07:1

Settin2 the bottom sear ratio (i_g1):

This ratio is dependent on the maximum tractive effort available in top gear, and the maximum resistance the vehicle is likely to encounter: maximum gradient + rolling resistance. The air resistance is neglected, since the vehicle speed to climb the maximum gradient is too low. The greatest gradient that is likely to be encountered is decided by the terrain the vehicle is to operate over. This is normally means a maximum gradient of 5 to 1 and in extreme 4 to 1.

* A safety factor has to be employed which is necessary due to the fall in engine performance during the engine’s working hfe, and as a safeguard in cases of poor performance due to maladjustment of ignition or carburetion and the like. This safety factor is usually applied after intermediate ratios have been selected and the bottom ratio is moved out of geometrical progression.

The torque required at the wheel (T_w) is equal to the resistance torque (T_TR), AR is neglected at low car velocity.

T_w = T_TR = (RR + GR ) x R_w

The torque at the wheel in this case is equal to:

Then; Tw = Tmax ig if ηt Tmax ig if ηt = (RR+GR) Rw ig = [(RR + GR) Rw] / (Tmax if ηt) where:          RR is the rolling resistance GR is the maximum gradient resistance the car can climb Rw is the effective tire radius ηt is the total transmission efficiency at the bottom gear if is the final drive ratio (obtained from the previous section)

Worked example:

A vehicle weighting 1500 kg has a coefficient of rolling resistance of 0.015. The transmission has a final drive ratio 4.07: 1 and an overall mechanical efficiency of 85%.

If the engine develops a maximum torque of 100 Nm and the effective road wheel radius is 0.27 m, determine the gearbox bottom gear ratio, if the vehicle to climb a maximum gradient of 4:1.

Solution:

RR = f_rW =f_r mg = 0.015 x 1500 x 9.81 = 220.725 N

GR = W sin θ =(mg) sin θ = 1500 x 9.81 x (1/4) = 3678.75 N

TR = RR + GR = 220.725 + 3678.75 = 3899.475 N

i_g = [(RR + GR) R_w] / ( T_max i_f η_t)

i_g = [3899.475 x 0.27] / (100 x 4.07 x 0.85) = 3.04: 1

Setting intermediate sear ratios

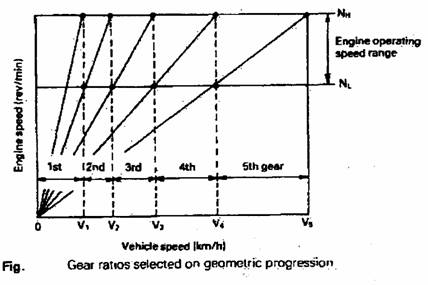
Ratio between top and bottom gears should be spaced in such a way that they will provide the tractive effort-speed characteristics as close to the ideal as possible. Intermediate ratios can be best selected as a first approximation by using a geometric progression. This method of obtaining the gear ratios requires the engine to operate within the same speed range in each gear, which is normally selected to provide the best fuel economy.

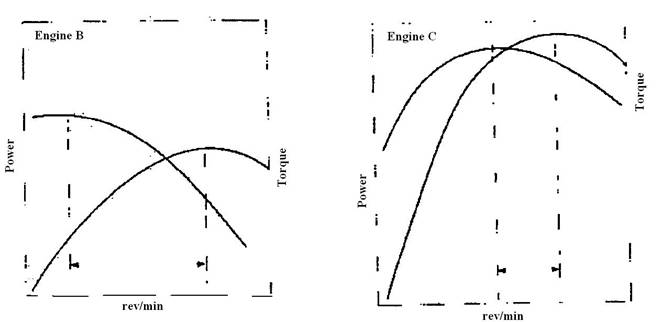
Before the intermediate ratios can be calculated a constant called the engine speed ratio has to be found. This is the ratio of engine re/mm at which maximum torque is generated to the rev/mm when maximum engine power is produced.

Engine speed ratio (K) = N_e at maximum torque / N_e at maximum power

The working engine speed range can be shown graphically and the larger the distance between point of maximum torque and point of maximum power rev/min, the greater the flexibility as seen in the figure. The engine with greater flexibility (engine B) needs a gearbox with fewer gear ratios.

* The engine working range (between maximum torque and maximum power) is suitable for car driving condition, when the car encounter additional minor resistance while moving, that will lead to decrease in car velocity (decrease in engine rpm) the reduction in engine rpm will lead to increase in the engine torque that will overcome this resistance, without the need to change to a lower gear. 

The engine speed ratio K is used to assist in the setting of the intermediate ratios once bottom gear has been calculated. This constant will provide ratios in a geometrical progression. This can be written as:

i_g2/i_g1 = i_g3/i_g2 = i_g4/i_g3 = i_g5/i_g4 = N_L / N_H = K

where:

i is the n gearbox ratio

N_L is the lower engine speed rev/mm,

N_H is the highest engine speed

i_g5/i_gl = (i_g2/i_g1)  (i_g3/i_g2)  (i_g4/i_g3)  (i_g5/i_g4) = (K) (K) (K) (K) = K⁴ = K^(n-1)

Where n is the number of gear shill. The gear ratios can be obtained from the above equation as follow:

i_g1 = i_g1 K^(1-1) = i_g1 K⁰ = i_g1  (the bottom gear ratio)

i_g2 = i_g1 K^(2-1) = i_g1 K

i_g3 = i_g1 K^(3-1) = i_g1 K²

i_g1 = i_g1 K^(4-1) = i_g1 K³

i_g1 = i_g1 K^(5-1) = i_g1 K⁴

For commercial vehicles, the gear ratios in the gearbox are often arranged in geometric progression. For passenger cars, to suit the changing traffic condition, the steps between the ratios of the upper two gears is often closer than that based on geometric progression. As a result, this will affect the section of the lower gears to some extent. In case the power to weight ratio is decreased, the number of gearbox shifts need to be increase, so we can get more surplus tractive effort to the acceleration.

After the intermediate ratios have been decided upon a safety factor may be placed on the bottom gear lowering it s ratio wise out of geometrical progression, and any manufacturing and design modifications can be applied.

Sitting intermediate gear ratios graphically:

Consider the engine to vehicle speed characteristics for each gear ratio as shown in the figure. When changing gear the engine speed will drop from the highest N_H to the lowest N_L without any change in the road speed i.e. v₁, v₂, etc.

Worked example:

A transmission system for a vehicle is to have an overall bottom and top gear ratio of 20:1 and 4.8 respectively. If the minimum and maximum speeds at each gear changes are 2100 and 3000 rev/mm respectively, determine the following:

a) the intermediate overall gear ratios b) the intermediate gear box and top gear ratios.

K=N_L/N_H= 2100 /3000 = 0.7

a)       Total 1^st gear ratio = (i_g1 i_f) = 20: 1

Total 2^nd gear ratio = (i_g1 i_f) K = 20 x 0.7 = 14.0: 1

Total 3^rd gear ratio = (i_g1 i_f) K² = 20 x 0.7² = 9.8:1

Total 4^th gear ratio = (i_g1 i_f) K³ = 20 x 0.7³ = 6.86: 1

Total 5^th gear ratio = (i_g1 i_f) K⁴ = 20 x 0.7⁴ = 4.8: 1

i_g1 = Total 1^st gear ratio/i_f = 20/4.8 = 4.166:1 (the bottom gear ratio)

i_g2 = Total 2^nd gear ratio/i_f = 14/4.8 = 2.916:1

i_g3 = Total 1^st gear ratio/i_f = 9.8/4.8 = 2.042:1

i_g4 = Total 1^st gear ratio/i_f = 6.86/4.8 = 1.429:1

i_g5 = Total 1^st gear ratio/i_f = 4.8/4.8 = 1:1 (top gearbox ratio and direct drive)

Setting the Gearbox and Final Drive Ratios

Sitting intermediate gear ratios graphically:

Consider the engine to vehicle speed characteristics for each gear ratio as shown in the figure. When changing gear the engine speed will drop from the highest N_H to the lowest N_L without any change in the road speed i.e. v₁, v₂, etc.

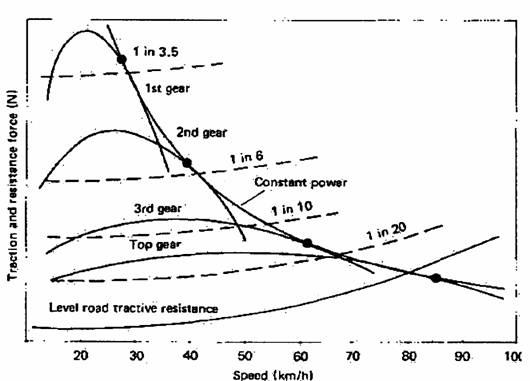
In the figure below a method of showing the engine’s speed ratio is given. This is based on the assumption that engine revolutions should not fall below the speed of maximum torque. The point at which the top gear line crosses the maximum torque line is the point at which a gear change should be mad. The change is indicated by a vertical line ab, and the line drawn from b to the origin 0 represents the conditions after the change down. The process is repeated for the other ratios. The horizontal distance between the vertical lines increase in geometrical progression or (approximately to this) depending upon whether modifications to some or all the calculated ratios has been made.

top gear = 3200/3200 = 1 to 1

3^rd gear = 3200/ 1800 = 1.77 to 1

2^nd gear = 3200/1100 =2.91 to 1

1^st gear = 3200/740 = 4.325 to 1

Engine speed ratio = 1800 / 3200 = 0.5625

* Note that in the above gear settings, after doing the calculations the first gear has been lowered slightly out of geometrical progression with second, and third gear moved closer to top gear, hence the speed ratio 0.5625 would not now apply.

Worked example (calculating the possible gear ratios for a vehicle):

Calculate the gear and final drive ratios for a vehicle from the given data:

Maximum engine torque is 105.2 N m at 2100 rev/mm.

Maximum power is 37.3 kW at 4000 rev/mm,

giving a road speed of 130 km/h obtained from graphs and data.

The rolling radius of road wheels is 0.366 m.

Transmission efficiency is 90%

and the maximum tractive resistance is 4890 N.

V = 0.377 R_w N_w = 0.377 R_w N_e/ (i_g i_f)

V_max = 0.377 R_w N_{w max} = 0.377 R_w N_e(pmax) /( i_g i_f)

i_f = 0.377 R_w N_e(pmax) /( i_g V_max) = 0.377 x 0.366 x 4000/(1 x 130) = 4.245 : 1 (final drive)

* maximum tractive resistance (TR_max) = maximum tractive effort at bottom gear

                   TR_max = T_{e max}  i_g  i_f η_t / R_w

i_g = TR_max R_w / (T_{e max} i_f η_t) = 4895 x 0.366 / (105.2 x 4.245 x 0.9) = 4.458 : 1

* engine ratio (k) = N_{e (max torque)} / N_{e (max power)} = 2100/4000 = 0.525

                   i_g1 = 4.458 :1

                   i_g2 = K i_g1 = 0.525 x 4.458 = 2.34 : 1

                   i_g3 = K² i_g1 = 0.525² x 4.458 = 1.228 : 1

                   i_g4 = K³ i_g1 = 0.525³ x 4.458 = 0.645 : 1  ≈ 1 : 1