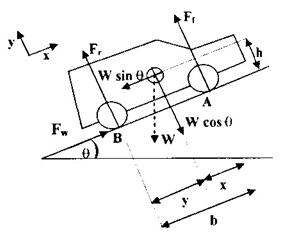
Vehicle Characteristics
VEHICLE DIMENSIONS:
 |
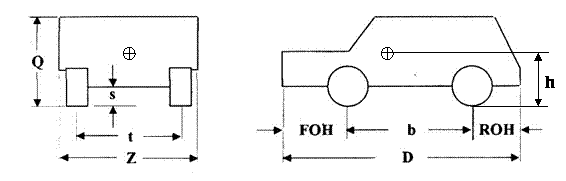t = Track D = Vehicle length
Q = Vehicle height b = Wheel base s = Ground clearance
Z = Vehicle width FOH = Front overhanging ROH = Rear overhanging
* h = the height of the car center of gravity (CG)
A) VEHICLE AXLES WEIGHTS: stationary
S MB = 0
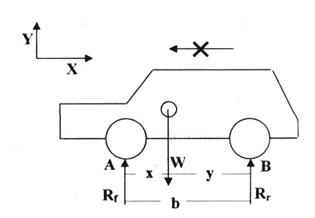
Rf . b – W . y = 0
Rf . b = W . y
Rf = W . y / b
S Fy = 0
Rf + Rr – W = 0
Rr = W - Rf
= W – W.y/b
= W.b/b – W.y/b
= W.(b-y)/b
Rr = W . x / b
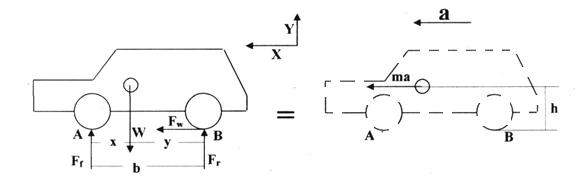B) VEHICLE AXLES WEIGHTS: acceleration
(1st method)
S
MB = 
Mb’
Ff . b + W . y = ma . h
Ff . b – W. y = - ma . h
Ff . b = W. y - ma . h
Ff = W. y/b - ma . h/b
Ff = Rf – wt
S Fy = Fy’
Fr + Ff – w = 0
Fr = W - Ff
Fr = W –(Rf – wt) = W – Rf + wt
Fr = Rr + wt
S Fx = Fx’
Fw = ma
Where
Ff = weight on the front axle due to weight and inertia force (car speed changes)
Fr = weight on the rear axle due to weight and inertia force (car speed changes)
Rf = static weight on the front axle (car is stationary)
Rr = static weight on the rear axle (car is stationary)
wt = transferred weight (ma . h/b)
ma = an imaginary force due to acceleration, in the direction of acceleration
Fw = the wheel force to accelerate the car
(2nd method)
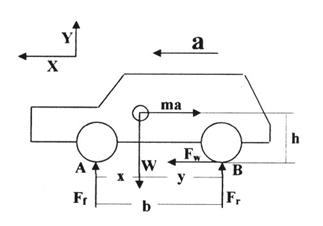(Regarding the inertia force as a
real force in the opposite direction of the acceleration)
S
MB = 0
Ff . b + W . y + ma . h = 0
Ff . b - W . y + ma . h = 0
Ff . b = W. y - ma . h
Ff = W. y/b - ma . h/b
Ff = Rf – wt
Where:
ma = a force due to inertia in the opposite direction of acceleration = Fw
S Fx = 0
Fr + Ff – W = 0
Fr = W – Ff = W – (W y/b – ma . h/b) = W. x/b + ma .h./b
Fr = Rr + wt
S Fy = 0
Fw – ma = 0
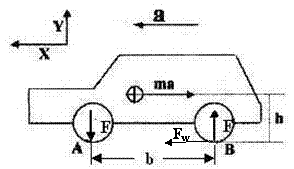(3rd method)
(Effect of the inertia force only)
S
MB = 0
F . b + ma . h = 0
F . b + ma . h + = 0
F . b = ma . h
F = ma . h/b
Where F is a force increases the reaction of ground on the front axle and decreases the reaction of the rear axle. This force is due to inertia force and is called the transferred weight “wt”. Then this force will be added to the stationary car front axle weight, and will be subtracted from the stationary car rear axle weight.
F = wt = ma h/b
Ff = Rf + wt,
Fr = Rr – wt,
Fw = ma
* During acceleration the rear axle load increases and the front axle decreases by the same amount (transferred weight). The transferred weight (wt) depend on the car mass (m) and the value of acceleration (a) and the height of CG (h) and wheel base (b).
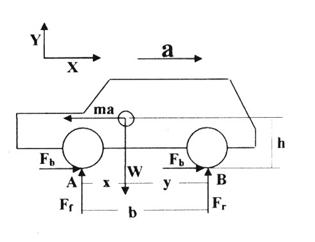
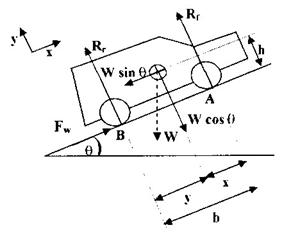
C) VEHICLE AXLES WEIGHTS: deceleration
S
MB = 0
Ff . b + W . y + ma . h = 0
Ff . b – W. y - ma . h = 0
Ff . b = W. y + ma . h
Ff = W. y/b + ma . h/b
Ff = Rf + wt
Where:
ma = a force due to inertia in the opposite direction of acceleration = Fw
S Fx = 0
Fr + Ff – W = 0
Fr = W – Ff = W – (W y/b + ma . h/b) = W. x/b - ma .h./b
Fr = Rr - wt
S Fy = 0
Fb – ma = 0
Fb = ma
Where: Fb is the braking force
Transferred weight (wt)
A vehicle under acceleration due to driving has the tractive force (Fw) introduced between tires and road surface of the driving axle, and at the same time an equal but opposite inertia force (ma) acts at the point of centre of gravity crating a moment (ma h) which transfers weight (wt) or vertical loading from front to rear axle (wheels).
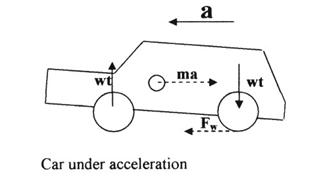
A vehicle under retardation due to braking has the frictional forces (Fb) introduced between tires and road surface, and at the same time an equal but opposite inertia force (ma) acts at the point of center of gravity creating a moment (ma h) which transfers weight (wt) or vertical loading from rear to front wheels.
From the above it can be seen that the value of the transferred weight is dependent upon the value of the inertia force Fi (ma) and the dimensions h and b. For a given acceleration or retardation the value of the weight transferred can be reduced by either increasing the wheelbase or reducing the height of the centre of gravity, or combination of both.
Since the adhesion force between tire and road depends on the weight of the tire, as will as the coefficient of adhesion, then; the transferred weight will affect the value of maximum tractive effort and maximum braking force. The weight on the driving axle will affect the value of maximum acceleration and maximum gradient for the car. The weight on the braking axles will affect the wheel lockup condition of the car wheels.
MAXIMUM POSSIBLE ACCELERATION (no wheel spin): level road
* The limit for the wheel spinning is Fw = m Rw
Rear wheel drive
Maximum acceleration force
Fw = m (static loading + transferred weight
= m (Rr + wt)
where
wt = ma h/b
thus
(Fw)max = m (Rr + m amax h/b)
from S Fx = 0
(Fw)max = m amax
then
m amax = m Rr + m m amax (h/b)
m amax - m m amax (h/b) = m Rr
m amax (1-m (h/b)) = m m g (x/b)
and maximum acceleration
amax = [m g (x/b)] / [1-m (h/b)] = m g x / (b- m h)
If the wheel or axle loading is given as a proportion or percentage of the vehicle weight (%) the above formula can be modified as follows:
amax = m g % / (1- m (h/b)) . . . . . . . .{spin limit}, or
amax = m g (x/b) / ((b- m h )/b) = [m x / (b- m h)] . g
* The limit for the car to flip over is when the transferred weight is equal to the load on the front axle of the car (Ff = 0).
m (amax)limit (h/b) = m g (y/b)
(amax)limit = g (y/h) . . . . . . . . . . . . . {flip over limit}
* The limit for the car engine torque is when the wheel torque at its maximum value.
m amax = Fw = (Tw)max / Rw = (Te)max. ig . if / Rw
amax = (Te)max. ig . if / (Rw .m). . . . . .{engine torque limit}
Traction control system TCS (ASR)
Wheel spin can be encountered under a variety of conditions: During operation on slippery road surfaces affording only limited traction on one or both sides, while exiting ice-covered parking lots and road shoulders, during acceleration when cornering, or when accelerating from rest on steep slopes. When spinning- as when locked- the wheel provides only a limited amount of lateral adhesion (instability). Freely spinning wheels also cause wear in both tires and driveline components (such as the differential), with severe loads occurring when the wheel suddenly grabs when it hits a high-traction surface. TCS ensures optimal application of tractive forces by preventing wheel spin. TCS is an extension of ABS, with which it shares numerous components. The force which can be transferred when starting off or during acceleration is (as for braking) a function of the slip between tire and road surface. When braking, a wheel will lock within a few tenths of a second, while build-up of excess torque during acceleration leads to a rapid increase in the rotational speeds of one or both drive wheels. TCS actually performs two functions: it enhances traction while maintaining vehicle stability (vehicle stays in lane).
Design
Although TCS uses the same components as the ABS, some of these have had extra functions added see the figure.
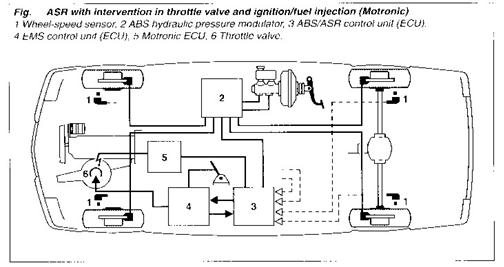
Wheel-speed sensors
The wheel-speed sensors provide the ECU with signals which enable it to calculate the
peripheral wheel speeds.
ECU
The ABS electronic circuitry has been extended by the addition of a TCS stage. The same as with ABS, the signals from the wheel-speed sensors are applied to the ECU input circuits. Here, they are used to calculate the wheel slip of the individual wheels, and TCS intervention is initiated if the slip at one of the wheels is found to be excessive. The incoming signals are processed in two micro controllers operating in parallel, and are converted in the output circuit into positioning commands for the solenoid valves and for the hydraulic-modulator supply pump which are responsible for implementing the brake-torque control. Information for engine management (Motronic) is transferred to ECU via an additional interface.
Hydraulic modulator
A TCS stage has been added to the ABS hydraulic modulator. It implements the orders from the ECU and, independent of the driver, controls the individual hydraulic pressures at the wheel brakes via the solenoid valves. During a single TCS control process, a supplementary pilot valve switches from the conventional braking mode to the TCS mode. The ABS return pump draws brake fluid from the master cylinder and generates the TCS system pressure. This means that braking pressure can be applied to the driven-wheel brake cylinders without any action on the part of the driver.
Wheel brakes
The braking pressure applied by the hydraulic modulator to the wheel-brake cylinders serves to force the brake linings against the brake drums or brake discs.
Compressed-air systems for commercial vehicles
Legal requirements
Effective as of 1 January, 1994, trucks within a GVW of 12 tons and above, and buses from 10 tons upward, must be equipped with a road-speed limitation facility. Maximum speed is 85 km/h for trucks and 100km/h for buses. The ABS/ASR-M and ABS/ASR-P systems satisfy these requirements.
Control concept
Brake control circuit
When the road surface provides different levels of traction on the left and right sides, it will usually be only the wheel on the low-traction surface that spins. Under these circumstances it frequently proves impossible to transmit the required tractive force to the road, and the vehicle fails to pull away.
The wheel-speed-sensor signals received by the ECU enable it to recognize impending wheel spin. It reacts by triggering the wheel's solenoid valve, so system pressure is directed to the pressure-control valve via the shuttle valve. The pressure-control valve relays modulated pressure to the brake cylinder, metering the pressure to allow the braking torque at the high-traction wheel to serve as drive (tractive) torque. In other words, ASR functions as an automatic limited-slip differential. During initial acceleration from a standing start, the brake controller operates up to a speed of 30 km/h. When the wheels lose traction at speeds in excess of 30 km/h, responsibility for corrective action reverts from the ASR brake-control circuit to the closed-loop engine-control circuit.
Closed-loop engine-control circuit
During standing start and acceleration, based on vehicle speed and the velocities of the drive wheels themselves, the ECU detects incipient loss of traction at the drive wheels and responds to this condition by reducing engine torque to the ideal level.
The ECU can apply various strategies for engine intervention. Potential actuators, or final
controlling elements, include:
- Electronic throttle control (ETC),
- Electronic diesel control (ETC),
- Proportioning valve with positioning cylinder P, Fig. 2,
- Servomotor M, Fig. 1, and
- Linear actuator.
When systems M and P are employed, the ECU directly reduces the drive torque at the diesel engine's fuel-injection pump by means of a servomotor or a valve with positioning cylinder. The other concepts rely on a reduction signal transmitted to the engine-management system. The TCS indicator lamp informs the driver of active TCS operation. This means that it functions as slip-warning indicator.
On gasoline engines the drive torque is controlled by the ECU for ETC (drive-by-wire) or the ECU for Motronic with integrated ETC. The following components/systems are influenced in
- Throttle-valve setting (adjusted by ETC).
- Ignition system (ignition timing adjusted by Motronic).
- Fuel-injection system (suppression of individual fuel-injection and ignition signals by Motronic).
MAXIMUM POSSIBLE DECELERATION (no wheel skid)
* The limit for wheel skidding is (Fb)w = m (R)w
MAXIUM POSSIBLE GRADIENT
The gradient effect on the car:
UP hill:
1- Increase the car motion resistance; FG = W sin q (against the direction of motion(
2- Increase the load on rear axle and decrease the load on the front one.
3- Decrease the stopping distance when using the brakes (4% is equal to 0.04 g(
Down hill:
1- Increase the tractive effort, FG = W sin q (in the direction of motion)
2- Increase the load on front axle and decrease the load on the rear one.
3-
Increase the stopping distance when
using the brakes (4% is equal to 0.04 g).
VEHICLE AXLE WEIGHT
a) Up hill
Ff = Rf - wt, and
Fr = Rr + wt
Where:
Ff, Fr are the reaction force on the front and
rear axles respectively.
Rf, Rr are the static reaction on the front and
rear axle respectively when the car on a level road.
Rf = W (cos q) y/b, Rr = W (cos q) x/b
wt is the weight transferred from higher axle on the gradient to the other axle.
wt = W (sin q) h/b
Maximum gradient the car can climb (constant velocity)
A- Rear wheel drive
(neglecting the air and inertia force due to acceleration or retardation)
S MA = 0
(W cos q) . x + (W sinq) . h – Rr b = 0 ……….(A-1)
S Fx = 0
Fw = W sin q
But: (Fx)max = Rr = W sin q
At (Fx)max Rr = (W sin q)/m ……….(A-2)
Substituting Eq. (A-1) into Eq. (A-2)
(W cos q) . x + (W sinq) . h – [(W sin q)/m ] . b = 0 ………(A-3)
Dividing Eq. (A-3) by (W cos q)
x + (tan q) . h – (tan q) (b/m) = 0
x = (tan q) (b/m) - (tan q) . h = (tan q) [(b-h . m)/m]
then
tan
q = 
……………………….(A-4)*
Taking the rolling resistance into account (RR = W fr) Eq. (A-4) becomes:
tan
q = 
……………………….(A-5)*
* the q limit in these equations are the car overturn (tan q = y/h).
Exercise:
1- For a front wheel drive car (FWD):
i- Obtain the equation of maximum acceleration
ii- Obtain the equation of maximum gradient
2- A front wheel drive (FWD) has a mass [m] of 1200 kg (the coefficient of adhesion between the tire and road [m] is 0.7), and it has the following dimensions:
- the wheel base [b] = 2.40 m
- the CG of the car is located 0.80 m behind the front wheel [x].
- the CG height is 0.6 m from the ground [h].
Find:
a- the transferred weight in:
i- moving with acceleration of 1.6 m/s2 (on level road).
ii- moving down gradient (down hill) of 1 in 4 (with constant velocity).
a- the wheel loads in the following cases
i- case a-i
ii- case a-ii
c- the maximum:
i- acceleration the car can attain on this kind of road (FWD & RWD)
ii-gradient the car can climb on this kind of road (FWD & RWD)