Rivets

Introduction

Rivets are considered to be permanent fasteners.   Riveted joints are therefore similar to welded and adhesive joints.   When considering the strength of riveted joints similar calculations are used as for bolted joints.

Rivets have been used in many large scale applications including shipbuilding, boilers, pressure vessels, bridges and buildings etc.   In recent years there has been a progressive move from riveted joints to welded, bonded and even bolted joints   A riveted joint, in larger quantities is sometimes cheaper than the other options but it requires higher skill levels and more access to both sides of the joint

There are strict standards and codes for riveted joints used for structural/pressure vessels engineering but the standards are less rigorous for using riveted joints in general mechanical engineering.

A rivet is a cylindrical body called a shank with a head.   A hot rivet is inserted into a hole passing through two clamped plates to be attached and the head is supported whilst a head is formed on the other end of the shank using a hammer or a special shaped tool. The plates are thus permanently attached. Cold rivets can be used for smaller sizes the - forming processes being dependent on the ductility of the rivet material...

When a hot rivet cools it contracts imposing a compressive (clamping) stress on the plates. The rivet itself is then in tension the tensile stress is approximately equal to the yield stress of the rivet material

---

Strength of riveted joint

The notes below are assuming that the plate loads are withstood by the rivets.   In practice the loads are generally withstood by friction between the plates under the compressive force of the contracted rivets.   The calculations provided below are simplified but provide relatively conservative joint strength value.  There is still a need to complete fatigue assessments on joints when relevant

Joint Types

There are two basic types of axial riveted joint the lap joint and the butt joint.

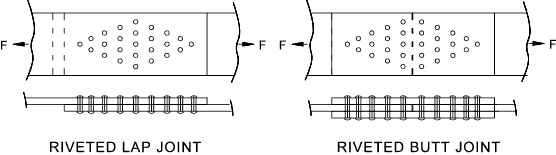
The selection of the number of rivets used for a joint and the array is simply to ensure the maximum strength of the rivets and the plates.  If ten small arrayed rivets on a lap joint were replaced by three large rivets across a plate the plate section area (in tension) would clearly be significantly reduced...

Rivet materials

Rivets for mechanical and structural applications are normally made from ductile (low carbon ) steel or wrought iron.   For applications where weight, corrosion, or material constraints apply, rivets can be made from copper (+alloys) aluminum (+alloys),monel etc.

Design stresses

For rivets used for structures and vessels etc the relevant design stresses are provided in the applicable codes. For rivets used in mechanical engineering, values are available in mechanical equipment standards which can be used with judgment.

BS 2573 Pt 1 Rules for the design of cranes includes design stress values based on the Yields stress (0,2% proof stress) Y_R0.2 as follows:-

Hand driven rivets ..tensile stress (40%Y_R0.2) ..Shear (36,6%Y_R0.2)..Bearing (80%Y_R0.2)

Machinery's handbook includes some values for steel rivets . I have interpreted these values and include them below as rough approximate values for first estimate.  These are typical values for ductile steel.   Tensile (76MPa) .. Shear (61MPa) ..Bearing (131MPa)

Design Assumptions

In designing rivet joints it is convenient to simplify the process by making the following assumptions.

• The rivets fail in either pure compression, or pure shear.
• The shear stress is evenly distributed across the rivet section.
• The bearing stress is evenly distributed across the projected area of the rivet.
• The force to cause a rivet to fail in double shear is 2 x the force to cause single shear failure.
• The tensile stress is uniform across the plate area between the rivets.

Rivet Joint Failure

A rivet joint may fail as a result of one (or more) of a number mechanisms..

• Shearing through one section of the rivet (single shear).
• Shearing through two sections of the rivet (double shear.
• Compressive bearing failure of the rivet.
• Shearing of the plate(s) being joined.
• Bearing failure of the plate(s) being joined.
• Tearing of the plates between the rivets.

Rivet Joint Efficiency

The rivet joint efficiency is simply described as follows

Eff = Max Allowable Force applied to Rivet Joint/ Plate Strength with no holes

The joint efficiency is increased by having multiple rows of rivets.  It is also clear that the efficiency can never be 100%.  The maximum allowable force is the smallest of the allowable shear, tensile or bearing forces.

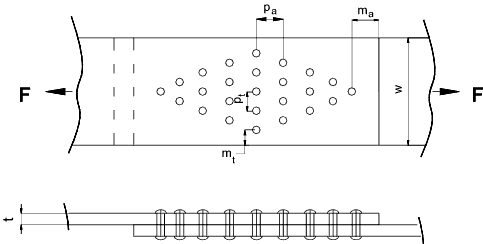
Rivets are initially sized with nominal diameters of between 1,2√ t and 1,4 √t (t = plate thickness)   The diametrical clearance provided for hot rivets is about 1,5 mm max.   For cold rivets very tight fits are often provided by using reamed holes.  It is important that the rivets are not positioned too close to the side of the plate or the edge of a plate. m _t should be greater than 1,5 d and m _a should be greater than 1,5 d. (d = nominal rivet diameter).   It is also suggested that the distance between rivets in the rows (p_t) is greater than 3d and the distance between rows (p_a) is greater than 3 d.  Using these guidance factors the strength calculations are simplified

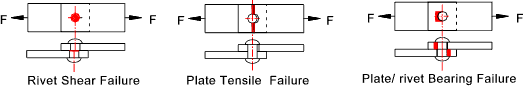
Rivets calculations are generally completed to check for three failure modes: rivet shear, plate tensile failure, and rivet /plate bearing. These are shown below.   A rivet joint can also fail due to plate shearing (tearing) behind the rivet.   This calculation is not always completed because the joint design should include that the minimum metal land behind the rivet (m_a above) is specified ensuring that other failure modes will operate before this mode.

It is also important that the axial pitch (p _a) is maximized (see above note) to ensure that the weakest section of the plate is through a row of holes

Rivet Shear
The rivet shear calculation is

τ = F_s / ( n p d ² /4 )

F_s = τ  ( n p d ² /4 )

• τ = Shear Stress (MPa)
• d = rivet diameter (mm)
• F_s = Total Axial Force (N)
• n = Number of Rivets

Plate Tensile Stress
The tensile stress in the plate =

σ_t = F_t / [t (w - n _r d ₁)]

F_t = σ_t [t (w - n _r d ₁)]

• σ_t = Tensile Stress (MPa)
• d ₁= rivet hole diameter (mm)
• n _r = Number of Rivets in a row across the plate
• w = plate width (mm)

Plate /rivet bearing stress

The Plate/Rivet bearing stress =

σ_c = F_c /(n d t)

F_c = σ_c (n d t)

• σ_c = Bearing Stress (MPa)
• d = rivet diameter (mm)
• n = Number of Rivets
• t = plate thickness (mm)

Plate tearing stress..

The plate shear stress =

τ_c = F /(2 m _a t)

Determine the rivet joint efficiency:

Eff = Max Allowable Force applied to Rivet Joint/ Plate Strength with no holes

1- Shearing efficiency of the rivets:

                                             Eff₁ = τ (n p d ² /4) / σ_t t w

                                        Eff₁ = τ (n p d ² /4) / σ_t t w                        (1)

2- Tensile efficiency of the plate:

Eff₂ = σ_t [t (w - n _r d ₁)] / σ_t t w

                                             Eff₂ = (w - n _r d ₁) / w                                   (2)

3- Crushing or bearing efficiency of the plate/rivets

                                              Eff₃ = σ_c (n d t) / σ_t t w

                                              Eff₃ = σ_c (n d) / σ_t w                                    (3)

* Note: The minimum efficiency of the rivet joint is the minimum efficiency in the above cases.